Thermodynamics and Thermochemistry: Hess’s Law, Gibbs Free Energy, and the Coefficient of Thermal Expansion

Hess’s Law of Constant Heat Summation

Definition

Hess’s law of constant heat Heat Inflammation summation, or Hess’s law, states that no matter how many steps or stages are present in a reaction, the total enthalpy Enthalpy Enzyme Kinetics change for the entire reaction is the sum of each individual change. Enthalpy Enthalpy Enzyme Kinetics is a thermodynamic measurement assessing the total heat Heat Inflammation content of a system (see image below). It is equivalent to the internal energy of the system plus the product of the pressure and volume:

H = U + pV PV Polycythemia vera (PV) is a chronic myeloproliferative neoplasm characterized by the overproduction of rbcs. In addition, the wbc and platelet counts are also increased, which differentiate pv from erythrocytosis seen with chronic hypoxia and other chronic conditions. Polycythemia Vera

Where:

H = enthalpy Enthalpy Enzyme Kinetics

U = internal energy

p = pressure

V = volume

In the reaction A → B → C, substance A is undergoing a reaction to become B, which then undergoes another reaction to become C. The change in enthalpy Enthalpy Enzyme Kinetics of the energy change is denoted by ΔH.

The ΔH for the total reaction is equal to the sum of the ΔH for the first reaction, plus the ΔH for the second reaction:

ΔH_{A͢͢͢͢͢ to B} + ΔH_{B to C} = ΔH_{A to C}

Calculations using Hess’s law

Example of the calculation that takes place in a reaction:

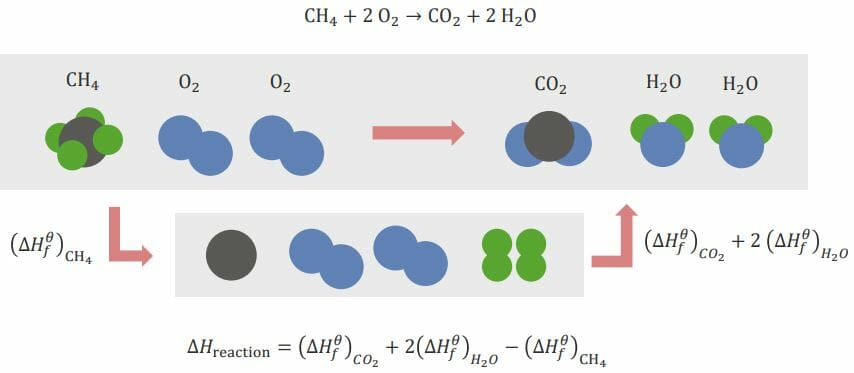
CH₄ + 2O₂ → CO₂ + 2H₂O

Here, methane and oxygen react to form carbon dioxide and water. The elements need to be rearranged before the reaction can occur. This is done by breaking existing bonds and forming new bonds between elements.

CH₄ (methane) needs to be broken down into its simple elements: C (carbon) and H (hydrogen). When this happens, an energy change occurs; this is referred to as (ΔH_f)_CH4. This is the standard energy of formation referring to standard elements present in nature. O₂ (oxygen) does not need to be broken down into individual O elements since, in nature, a single O isn’t present. The standard form is O₂.

To form the products, C and O have to combine to form CO₂ (carbon dioxide) and H and O have to combine to form H₂O (water). The enthalpies for forming these two products are (ΔH_f)_CO2 and (ΔH_f)_H2O, respectively.

The total enthalpy Enthalpy Enzyme Kinetics of the entire reaction is as follows:

ΔH_reaction = (ΔH_f)_CO2 + 2(ΔH_f)_H2O – (ΔH_f)_CH4

The minus sign for the enthalpy Enthalpy Enzyme Kinetics for methane is due to the break-up of the bonds that are taking place:

Hess’s law is used to:

1. Determine the heat Heat Inflammation of formation
2. Determine the heat Heat Inflammation of transition
3. Determine the heat Heat Inflammation of hydration
4. Calculate bond energies

Gibbs Free Energy 

Definition

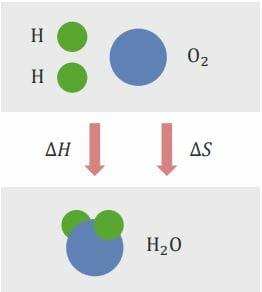
Gibbs free energy Free energy Enzyme Kinetics is the energy that is associated with a chemical reaction that can be used to perform work. It conveys the amount of free energy Free energy Enzyme Kinetics that exists for a reaction to go forward (see image below). In general, a reaction wants to minimize energy use ( enthalpy Enthalpy Enzyme Kinetics) and maximize entropy Entropy The measure of that part of the heat or energy of a system which is not available to perform work. Entropy increases in all natural (spontaneous and irreversible) processes. Enzyme Kinetics.

Entropy Entropy The measure of that part of the heat or energy of a system which is not available to perform work. Entropy increases in all natural (spontaneous and irreversible) processes. Enzyme Kinetics is the degree of disorder or randomness that exists in a system. The calculation of Gibbs free energy Free energy Enzyme Kinetics (ΔG) determines whether the reaction can occur spontaneously or not.

Enthalpy Enthalpy Enzyme Kinetics change (ΔH) and entropy Entropy The measure of that part of the heat or energy of a system which is not available to perform work. Entropy increases in all natural (spontaneous and irreversible) processes. Enzyme Kinetics change (ΔS) are competing reactions. Enthalpy Enthalpy Enzyme Kinetics seeks to be minimal, whereas entropy Entropy The measure of that part of the heat or energy of a system which is not available to perform work. Entropy increases in all natural (spontaneous and irreversible) processes. Enzyme Kinetics seeks to be maximal.

Entropy Entropy The measure of that part of the heat or energy of a system which is not available to perform work. Entropy increases in all natural (spontaneous and irreversible) processes. Enzyme Kinetics is also associated with temperature, since the disorder that exists in a system is dependent on temperature. The higher the temperature, the more disorder is present.

The relationship between Gibbs free energy Free energy Enzyme Kinetics (ΔG), enthalpy Enthalpy Enzyme Kinetics change (ΔH), and entropy Entropy The measure of that part of the heat or energy of a system which is not available to perform work. Entropy increases in all natural (spontaneous and irreversible) processes. Enzyme Kinetics change (ΔS) is as follows:

ΔG = ΔH – T ΔS

Where :

ΔG = Gibbs free energy Free energy Enzyme Kinetics

H = enthalpy Enthalpy Enzyme Kinetics

TS = random energy

ΔGsystem = –T ΔS total

Note the negative sign above; it denotes that enthalpy Enthalpy Enzyme Kinetics and entropy Entropy The measure of that part of the heat or energy of a system which is not available to perform work. Entropy increases in all natural (spontaneous and irreversible) processes. Enzyme Kinetics are opposing reaction concepts; as well, temperature (T) is measured using the Kelvin scale Scale Dermatologic Examination (K).

To Be Spontaneous or Not To Be Spontaneous; That is the Question

The reaction that needs to take place can occur either spontaneously (favorably) or nonspontaneously (unfavorably). This is determined by the calculation of Gibbs free energy Free energy Enzyme Kinetics (ΔG).

If ΔG < 0, then the reaction is considered to be a spontaneous reaction. If ΔG > 0, then the reaction is considered to be a non-spontaneous reaction.

Even if the reaction is exothermic (i.e., it requires no energy input), it may not occur if it lowers the entropy Entropy The measure of that part of the heat or energy of a system which is not available to perform work. Entropy increases in all natural (spontaneous and irreversible) processes. Enzyme Kinetics too much. As well, even if something is spontaneous, it does not necessarily occur quickly. Both of these concepts are not directly related, since they depend on different properties of the reaction.

Coefficient of Thermal Expansion

Definition

The coefficient of thermal expansion describes how the size of different types of matter can be affected (changed) by a change in temperature. Specifically, it measures the fractional change in size per degree change in temperature at a constant pressure. This change can be associated with linear (one-dimensional) change or volumetric (three-dimensional) change. When an object is heated (i.e., its temperature increases), the heated object is larger. Increasing heat Heat Inflammation will continue causing some expansion to occur based on the degree of Kelvin change in temperature.

Linear thermal expansion

An object has a linear dimension which, when heated, undergoes a linear expansion. The linear thermal expansion can only be measured in the solid state and is common in engineering applications. This change in thermal expansion in the linear (one-dimensional) direction is denoted as follows:

L_final = L_initial (1 + α ΔT)

Where:

L_final = final length

L_initial = initial length

α = coefficient of linear expansion

ΔT = temperature change

The coefficient of linear expansion is α = 1 / temperature; thus, the units for α are 1 / Kelvin.

Volumetric thermal expansion

An object has a volumetric dimension that, when heated, undergoes volumetric expansion. The volumetric thermal expansion can be measured for all condensed matter (liquid and solid states). This change in thermal expansion in the volumetric (three-dimensional) direction is denoted as follows:

Vfinal = Vinitial (1 + αv ΔT)

Where:

Vfinal = final volume

Vinitial = initial volume

αv = coefficient of volumetric expansion

ΔT = temperature change

The coefficient of volumetric expansion is αv = 3 α. This is because the volumetric coefficient is associated with three dimensions; thus, it is equal to three of the coefficient of linear expansions.

Simplifying thermal expansion calculations 

The thermal expansion equations for linear expansion and volumetric expansion can be stated in a different way based on a change in length and volume, respectively.

Linear expansion

L_final = L_initial (1 + α ΔT)

L_final = L_initial + (L_initial α ΔT)

L_final = L_initial + ΔL

Thus, the change in length is ΔL = L_initial α ΔT.

Volumetric expansion

V_final = V_initial (1 + α_v ΔT)

V_final = V_initial + (L_initial α_v ΔT)

V_final = V_initial + ΔL

Thus, the change in volume is ΔV = V_initial α_v ΔT.

Applications of thermal expansion include the following:

1. In mechanical applications to fit parts together
2. In applications using the thermal expansion property, such as in bi-metal and  mercury Mercury A silver metallic element that exists as a liquid at room temperature. It has the atomic symbol Hg (from hydrargyrum, liquid silver), atomic number 80, and atomic weight 200. 59. Mercury is used in many industrial applications and its salts have been employed therapeutically as purgatives, antisyphilitics, disinfectants, and astringents. It can be absorbed through the skin and mucous membranes which leads to mercury poisoning. Because of its toxicity, the clinical use of mercury and mercurials is diminishing. Renal Tubular Acidosis thermometers